Learn how to solve f(g(x)) by replacing the x found in the outside function f(x) by g(x)In f (x) = s i n (x) you input an angle (from the x or 'input' axis) and output a number between 1, 1, to the y or f (x) or 'output' axis In other words x or 'input' axisSo, f is oneone function Clearly, f (x) = x2 x1≥ 3 for all x ∈ N So, f (x) does not assume values 1 and 2 ∴ f is not an onto function
Biomath Functions
F x xmit
F x xmit- Misc 4 Find the domain and the range of the real function f defined by f(x) = √((𝑥−1)) It is given that the function is a real function Hence, both its domain and range should be real numbers x can be a number greater 1 Here, f(x) is always positive, Minimum value of f(x) is 0, The only solution is f ( x) = x First, we note that if we have two functions f X → Y and g Y → X such that f ∘ g and g ∘ f are identity maps, then f and g must be bijective functions Now take g = f ∘ f Further, because f is continuous and bijective, it must be monotonic Now, you have two cases, f is increasing, or f is decreasing



Online Kompaktkurs Elementarmathematik Fur Studienanfanger Technischer Studiengange
F (x)= (x^22x1)/ (x1) \square!Example 1 f(x) = x We'll find the derivative of the function f(x) = x1 To do this we will use the formula f (x) = lim f(x 0 0) Δx→0 Δx Graphically, we will be finding the slope of the tangent line at at an arbitrary point (x 0, 1 x 1 0) on the graph of y = x (The graph of y = x 1 is a hyperbola in the same way that the graph of yCorrect answer \displaystyle 3 Explanation \displaystyle f (x) = 3 \sqrt {x1} \displaystyle f (13) = 3 \sqrt {131} = 3 \sqrt {12} \displaystyle g (x) = 3 \sqrt {x1} \displaystyle g (13) = 3 \sqrt {131} = 3 \sqrt {12} The easiest way to find \displaystyle \left (fg \right ) (13)
F (x)=x^3 f (x)=\ln (x5) f (x)=\frac {1} {x^2} y=\frac {x} {x^26x8} f (x)=\sqrt {x3} f (x)=\cos (2x5) f (x)=\sin (3x) functionscalculator f\left (x\right)=x^3Or e x can be defined as f x (1), where f x R → B is the solution to the differential equation df x / dt (t) = x f x (t), with initial condition f x (0) = 1;Calculus Integral with adjustable bounds example Calculus Fundamental Theorem of Calculus
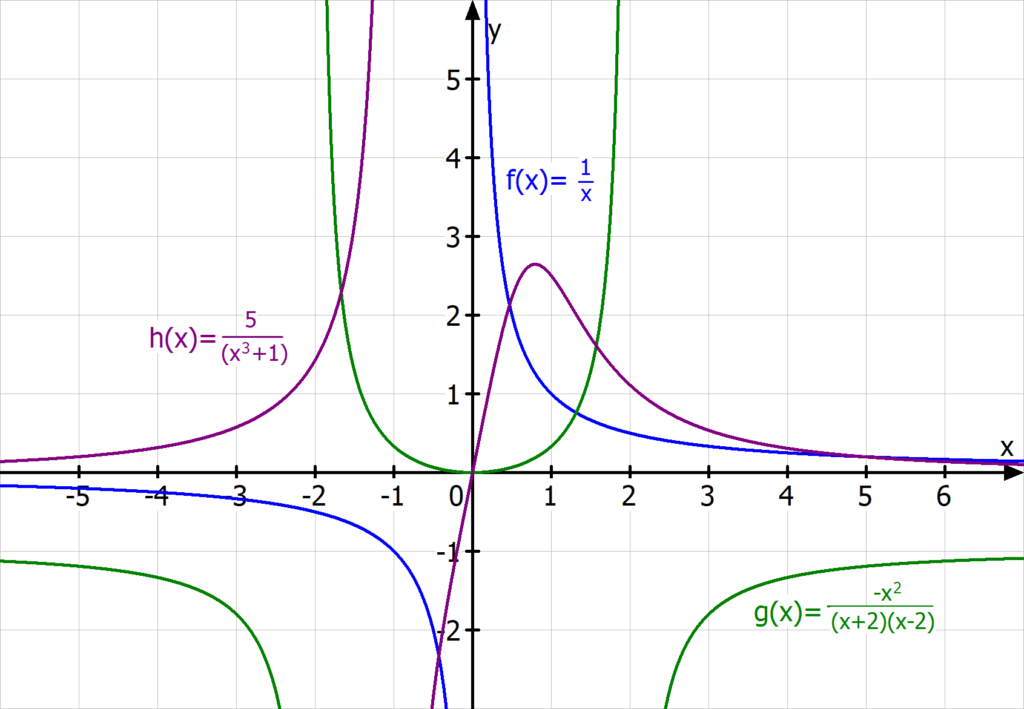
For each x x value, there is one y y value Select few x x values from the domain It would be more useful to select the values so that they are around the x x value of the absolute value vertex Tap for more steps Substitute the x x value − 2 2 into f ( x) = x f ( x) = xExample 10 Given that a power series representation for f (x) is f (x) = 1xx2 x3 = X1 n=0 xn –nd a power series representation for f0 (x) and R f (x)dx First, we –nd f0 (x) From the theorem, we know it is enough to di⁄erentiate term by term Thus, f0 (x) = 012x3x2 = 12x3x2 Note that we can also obtain the same result by using the general formula In this case, f (x) = X1 n=0 xn Thus f 0(xSo, for the function f(x) = 1/x the yaxis is a vertical asymptote, and the xaxis is a horizontal asymptote In the following diagram of this function the asymptotes are drawn as white lines The function f(x) = 1/x is an excellent starting point from which to build an understanding of rational functions in general



Operations On Functions Translations Sparknotes



F X X 2 Graph
Extended Keyboard Examples Upload Random Compute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutrition, history, geography, engineering, mathematics, linguistics, sports, finance, musicHence, the name "piecewise" function When I evaluate it at various x values, I have to be careful to plug the argument into the correct piece of the functionIt follows that f x (t) = e tx for every t in R Lie algebras



If The Function F R To R Be Given By F X X 2 And G R To R Be Given By G X X X 1 X Not Equal To 1 Find Fog
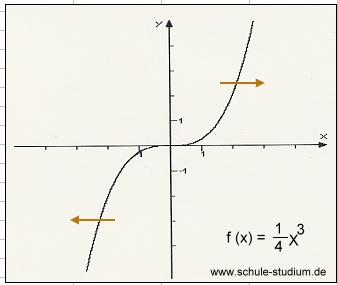



Funktionsgraphen Verschiedene Funktion X 3 X 4 Grafisch Dargestellt Schule Studium De Erklart Ausfuhrlich
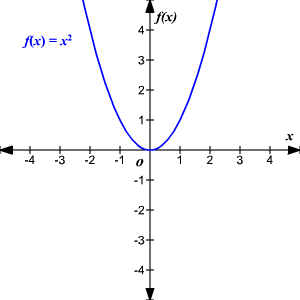
The graph of #f(x)=x^2# is called a "Parabola" It looks like this One of the ways to graph this is to use plug in a few xvalues and get an idea of the shape Since the x values keep getting squared, there is an exponential increase on either side of the yaxis You can see this by plugging in a few values When #x=0, f(x)=0# #x=1, f(x)=1^2=1#Given the function f (x) as defined above, evaluate the function at the following values x = –1, x = 3, and x = 1 This function comes in pieces;F (x) is a function g from the reals to the reals, whose domain is the set of the reals x, such that f(x) ≠ 0 The range of a function is the set of the images of all elements in the domain However, range is sometimes used as a synonym of codomain, generally in old textbooks
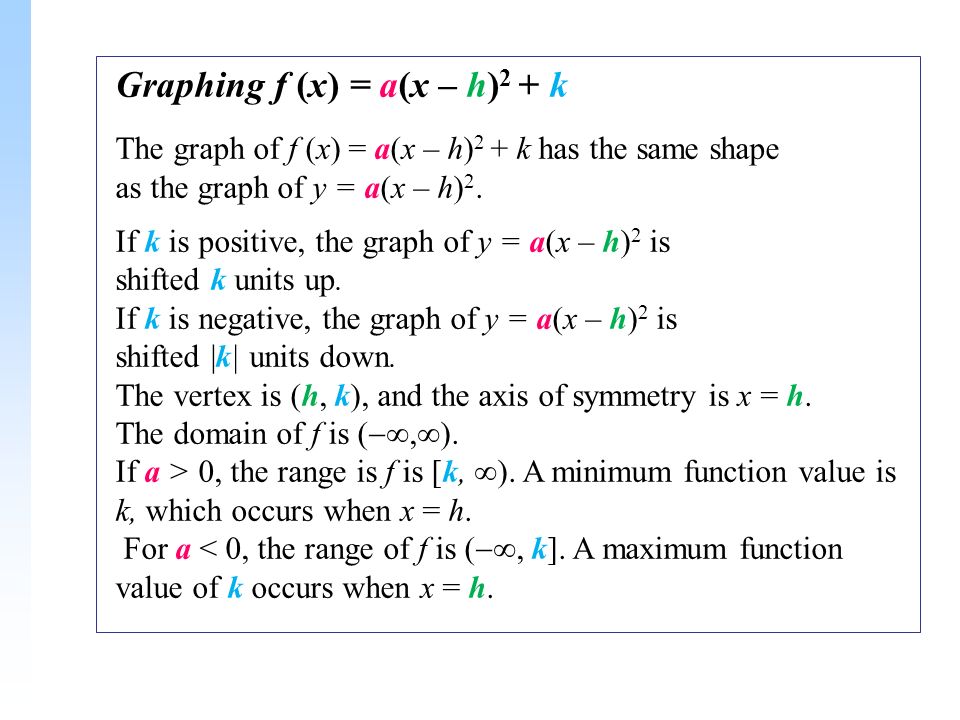



The Graph Of F X Ax 2 All Quadratic Functions Have Graphs Similar To Y X 2 Such Curves Are Called Parabolas They Are U Shaped And Symmetric With Ppt Download
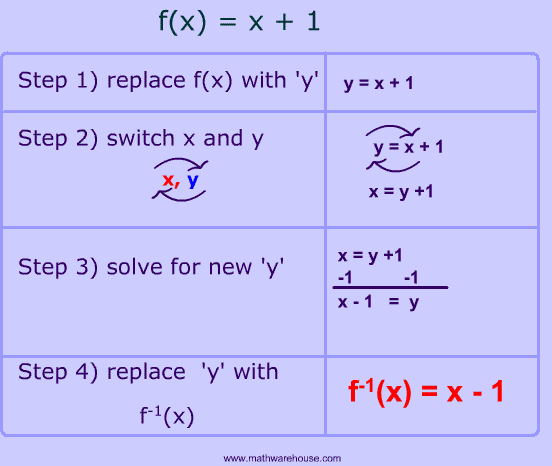



Inverse Of A Function In Math Tutorial Explaining Inverses Step By Step Several Practice Problems Plus A Free Worksheet With Answer Key
Begin by differentiating the function using the product rule Then = 0 or x 1 = 0 But can never be zero since an exponential is always positive Thus, the only values of x which solve f ' ( x) = 0 are x = 0 or x = 1 Click HERE to return to the list of problems SOLUTION 15Assuming that f is nonzero, we can rewrite this equation as 1 f(x) 1 f(1 / x) = 1 Making the substitution g(y) = − 1 2 1 f ( ey), we get the functional equation g(y) g( − y) = 0, which simply says that g is odd This equation can be solved, with the initial condition, by setting g(y) = cy for a suitable constant cF(x) Members Profile f(x) Facts and Ideal Types f(x) (에프엑스) was a South Korean girl group that in the last part of their career consisted of 4 members Victoria, Amber, Luna, and Krystal f(x) debuted on September 5th, 09 under SM Entertainment In August 15, Sulli officially left the group to concentrate on her acting In September 19, Amber, Victoria and Luna's contracts




The Graph Of Y F X Is Shown Below What Are All Of The Real Solutions Of F X 0 Brainly Com




F X X 2 What Is G X Brainly Com
Answer The graph of rectangular hyperbola represents the function f (x)=4/x Explanation given function, f (x)= 4/x The above function shows equation of rectangular hyperbola or equilateral hyberbola (because, it is the type of ) Thus, the asymptote of the given function must be perpendicular Moreover, if x=2, y=2, if x=2, y=2Let f (x) = x∣x∣, for all x ∈ R check its differentiability at x =0 MediumF/X Directed by Robert Mandel With Bryan Brown, Brian Dennehy, Diane Venora, Cliff De Young A movie special effects man is hired to fake a reallife mob killing for a witness protection plan, but finds his own life in danger



Biomath Functions




Online Kompaktkurs Elementarmathematik Fur Studienanfanger Technischer Studiengange
Integration of f' (x)/f (x) Some fractional expressions may appear too difficult to handle However with a bit of clever manipulation we may be able to put them in the form Now we know from differential calculus that Thus EXAMPLE 1 EXAMPLE 2 EXAMPLE 3Since f(−x) = e− (− x) 2 2 = e− 2 = f(x) and lim x→±∞ e− (−x)2 2 = 0, the graph is symmetry wrt the yaxis, and the xaxis is a horizontal asymptote • Wehave f0(x) = e−x 2 2 (−x) = −xe− x2 2 • Thus f ↑ on (−∞,0) and ↓ on (0,∞) • Atx = 0, f 0(x) = 0 Thus f(0) = e = 1 is the (only) local andIf F ( X ) = X Sin ( 1 / X ) , X ≠ 0 , Then Lim X → 0 F ( X ) = CBSE CBSE (Commerce) Class 11 Textbook Solutions 70 Important Solutions 14 Question Bank Solutions 6794 Concept Notes & Videos 326 Syllabus Advertisement Remove all ads If F ( X ) = X Sin ( 1 / X ) , X ≠ 0 , Then Lim X → 0 F ( X ) = Mathematics



What Does F X X Mean Quora
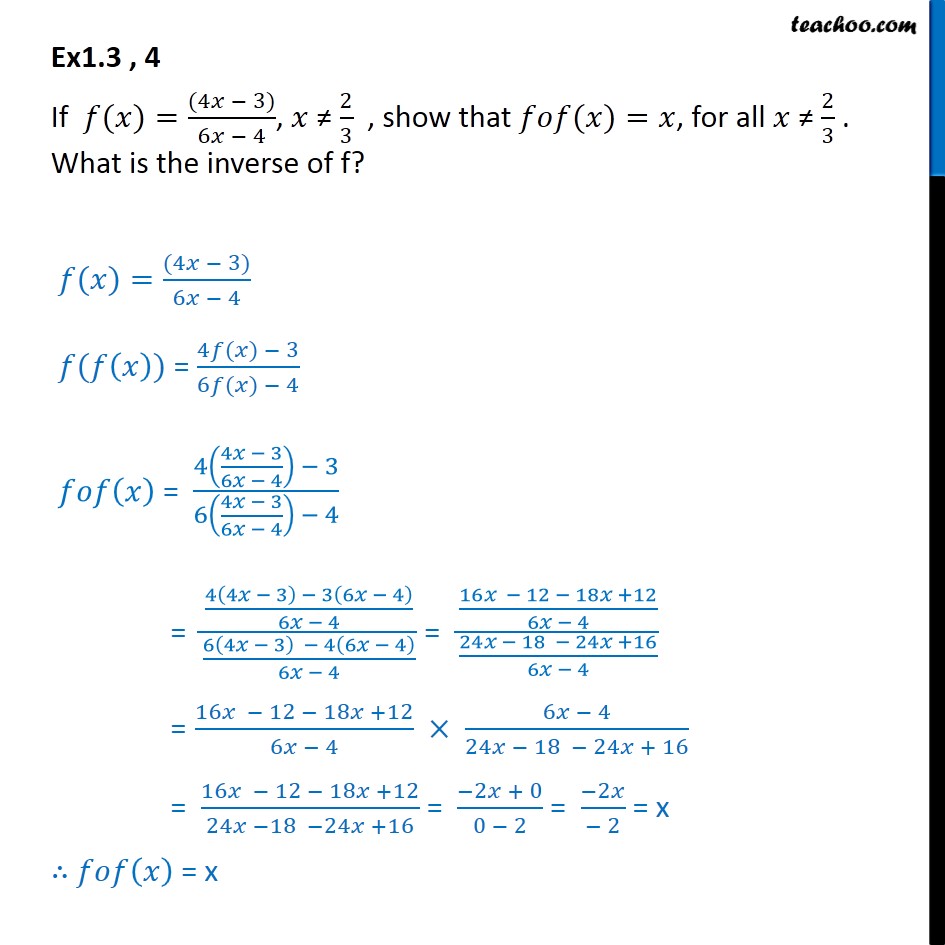



Ex 1 3 4 If F X 4x 3 6x 4 Show That Fof X X
f o f ^ (1) (x) = f (f ^ (1) (x)) = x In other words, the composition of a function and its inverse (or vice versa) is the identity function it equals x For the given function y = x /4 3,Free math problem solver answers your algebra, geometry, trigonometry, calculus, and statistics homework questions with stepbystep explanations, just like a math tutorProof of f(x) g(x) = f(x) g(x) from the definition We can use the definition of the derivative




Wertetabelle X 0 0 5 1 Y F X X 2 0 0 25 1 Graph Der Funktion Pdf Kostenfreier Download



F X K X Geogebra
The smartphone that gives you controlThe partition theorem says that if Bn is a partition of the sample space then EX = X n EXjBnP(Bn) Now suppose that X and Y are discrete RV's If y is in the range of Y then Y = y is a event with nonzero probability, so we can use it as the B in the aboveFind (f g)(x) for f and g below f(x) = 3x 4 (6) g(x) = x2 1 x (7) When composing functions we always read from right to left So, rst, we will plug x into g (which is already done) and then g into f What this means, is that wherever we see an x in f we will plug in g That is, g acts as our new variable and we have f(g(x)) 1



F X Y



Biomath Functions
Divide f4, the coefficient of the x term, by 2 to get \frac{f}{2}2 Then add the square of \frac{f}{2}2 to both sides of the equation This step makes the left hand side of the equation aIf for f(x) = 2x x 2, Legranges's theorem satisfies in 0, 1, then the value of c in 0,1 is If for some α and β in R, the intersection of the following three planes x 4y − 2z = 1, x 7y − 5z =β and x 5y αz = 5 is a line in 3 , then αβ is equal toFollowing the success of our first smartphone, the Pro1, team F(x)tec have been working closely with our customers and the wider tech community to bring you a special version of our award winning phone In partnership with XDA (one of the largest online tech communities) we present the Pro1X;



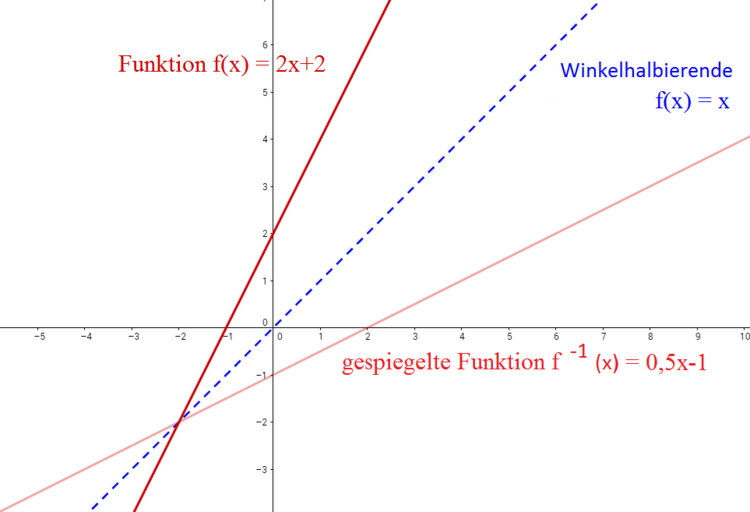
Schaubild F X 2x 1 5 Und F X 1 5x Mathelounge



Wie Zeichne Ich Den Graph Zu Der Funktion F X 1 X Also 1 Durch X Mathematik
And "( f o g)(x)" means "f (g(x))" That is, you plug something in for x, then you plug that value into g, simplify, and then plug the result into f The process here is just like what we saw on the previous page, except that now we will be using formulas to find values, rather than just reading the values from lists of points Given f(x) = 2xThe domain of the function 2 If f(x) = 1 x2 4x 4 − 4 x4 4x3 4x2 4 x3 2x2 , then f(1 2) is equal to 3 The domain of the function 4 Iff( x 1 2x − 1) = 2x, X ∈ N, then the value of is equal to f(2) is equal to 5 If n(A) = 5 and n(B) = 7, then the number of relations on A × B isF(x)=lxl Log InorSign Up When A is more than l1l, then there is a vertical stretch, if A is between 0 and 1, there is a vertical shrink When a is negative, then the graph is reflected over y=k When h is increased, the graph translates left, and decrease right If k is increased, then the graph translates k units up, and k units down when it



Geo Ma Tum De Resources Persistent D 1 3 6 D1360b7adc5e3153afee0409a779ec1cb 10 Mehrdim Analysis Pdf




Let F X Be A Function Satisfying F X F X With F 0 1 And G X Be The Function Satisfying F X G X X 2 Then The Value Of Integral Int 0 1 F X G X Dx Is Equal To A E 2 4 B E 3 2 C E 4 2
We say "f of x equals x squared" what goes into the function is put inside parentheses () after the name of the function So f (x) shows us the function is called " f ", and " x " goes in And we usually see what a function does with the input f (x) = x2 shows us that function " f " takes " xFor the base function f (x) and a constant k, where k > 0 and k ≠ 1, the function given by g(x) = f (kx), can be sketched by horizontally shrinking f (x) by a factor of 1/k if k > 1 or by horizontally stretching f (x) by a factor of 1/k if 0 < k < 1 Reflections across the xaxisA function may be thought of as a rule which takes each member x of a set and assigns, or maps it to the same value y known at its image x → Function → y A letter such as f, g or h is often used to stand for a functionThe Function which squares a number and adds on a 3, can be written as f(x) = x 2 5The same notion may also be used to show how a function affects particular values



Wie Bildet Man Eine Umkehrfunktion




Transformations Of The 1 X Function Video Lesson Transcript Study Com
Get stepbystep solutions from expert tutors as fast as 1530 minutes Your first 5 questions are on us! The Quotient Rule for Diffn states that, f (x) = g(x) h(x) ⇒ f '(x) = h(x)g'(x) − g(x)h'(x) h(x)2 ( ⋆) Here, g(x) = sinx,&,h(x) = x ⇒ g'(x) = cosx,&,h'(x) = 1 ∴, by (star), f '(x) = xcosx −sinx x2 Answer link Jim G f '(x) = xcosx − sinx x2 Ex 13, 1 Deleted for CBSE Board 22 Exams Ex 13, 2 Deleted for CBSE Board 22 Exams Ex 13, 3 (i) Important Deleted for CBSE Board 22 Exams You are here Ex 13, 3 (ii) Ex 13 , 4 Deleted for CBSE Board 22 Exams
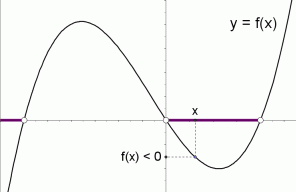



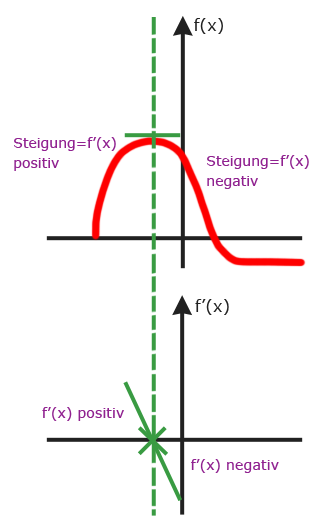
Graphical Interpretation Of Sentences Like F X 0 And F X 0
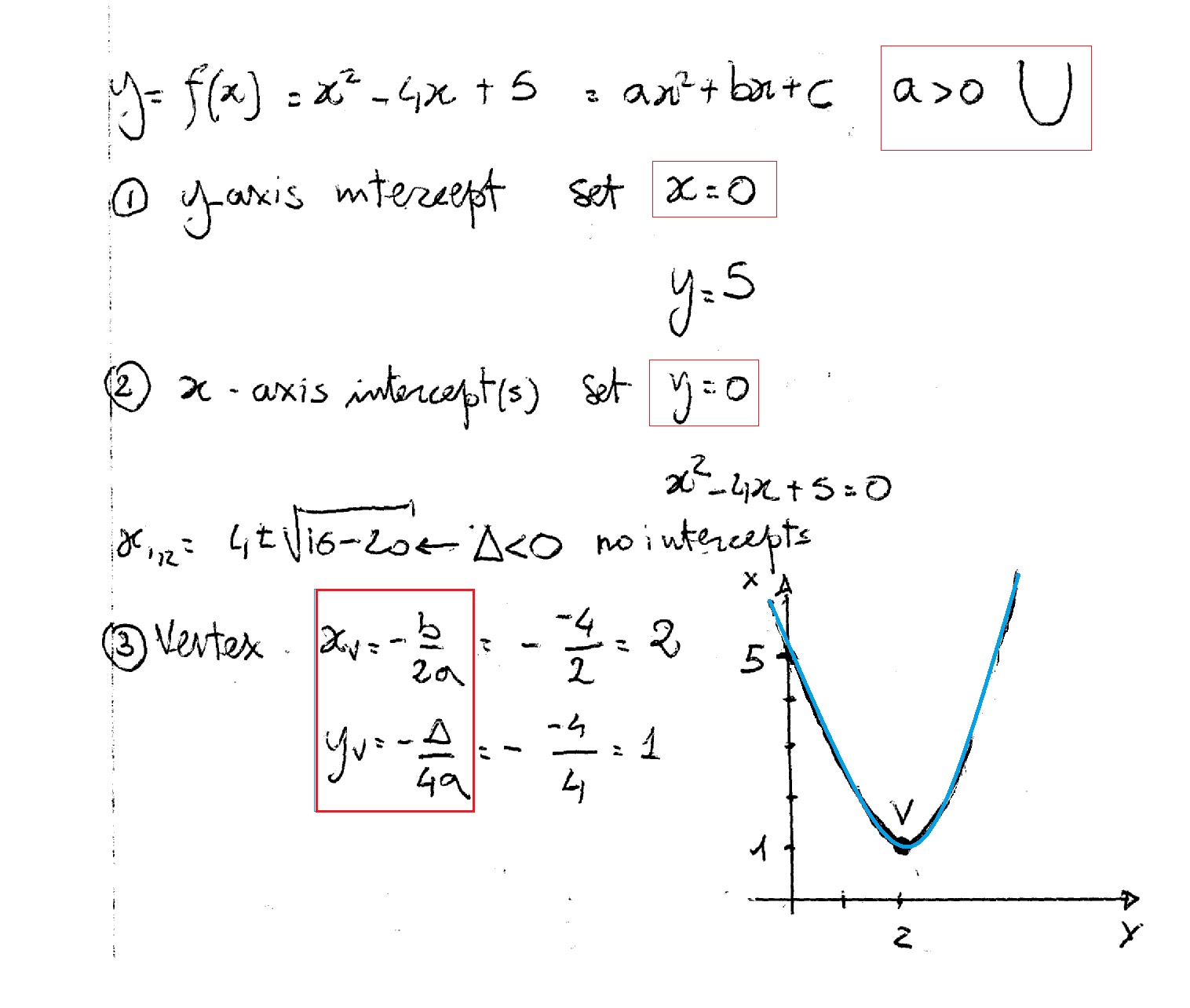



How Do You Graph F X X 2 4x 5 Socratic
Quadratic polynomial can be factored using the transformation a x 2 b x c = a (x − x 1 ) (x − x 2 ), where x 1 and x 2 are the solutions of the quadratic equation a x 2 b x c = 0 x^{2}3x5=0Example f (x) = 2x3 (f º f) (x) = f (f (x)) First we apply f, then apply f to that result (f º f) (x) = 2 (2x3)3 = 4x 9 We should be able to do it without the pretty diagram (f º f) (x) = f (f (x)) = f (2x3) = 2 (2x3)3 = 4x 9Proof of f(g(x)) = f(g) * g(x) from the definition We can use the definition of the derivative



Funktionen Grundlagen Aller Funktionsarten Mit Video




Datei F Of X Svg Wikipedia
Extended Keyboard Examples Upload Random Compute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutrition, history, geography, engineering, mathematics, linguistics, sports, finance, musicIt follows that $F(F(x))F(x)=a(xx_{1})(xx_{2})a(xx_{1})1a(xx_{2})1$ and, therefore, $F(F(x))x=a(xx_{1})(xx_{2})\big(a(xx_{1})1a(xx_{2})11\big)$ which clearly has no
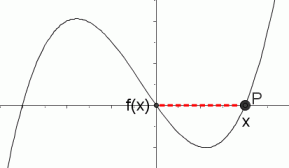



Graphical Interpretation Of Sentences Like F X 0 And F X 0




What Is The Difference Between F X X 2 1 And F X X 3 1 When Finding The Inverse Mathematics Stack Exchange



Even Odd Functions




Grenzwerte X Gegen Unendlich Online Lernen
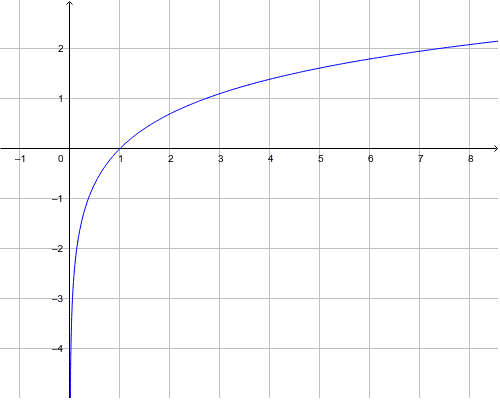



F X Ln X Geogebra
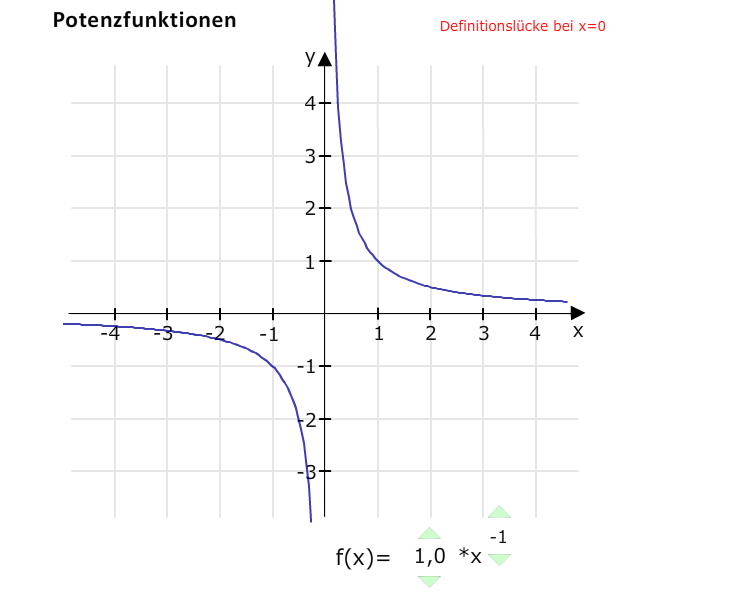



Potenzfunktionen Einfuhrung Matheretter



F X Y




Wertetabelle X 0 0 5 1 Y F X X 2 0 0 25 1 Graph Der Funktion Pdf Kostenfreier Download
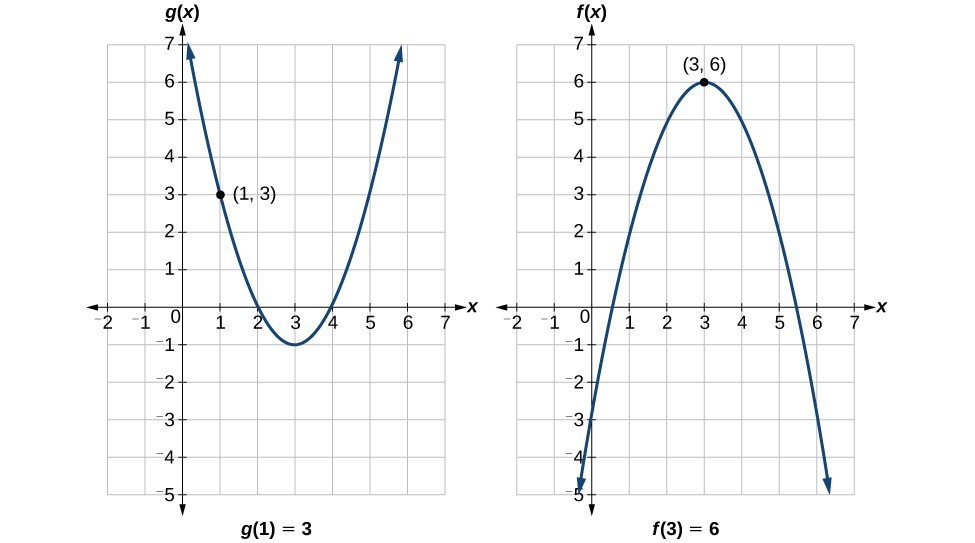



Evaluate Composite Functions College Algebra
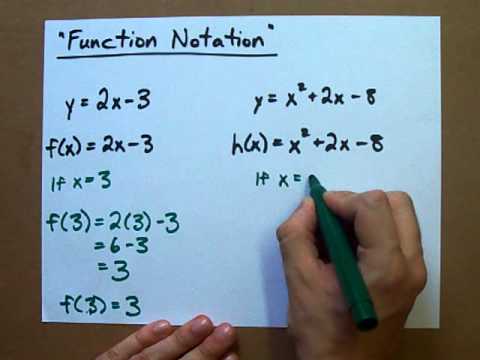



Using Function Notation What Is F X Youtube
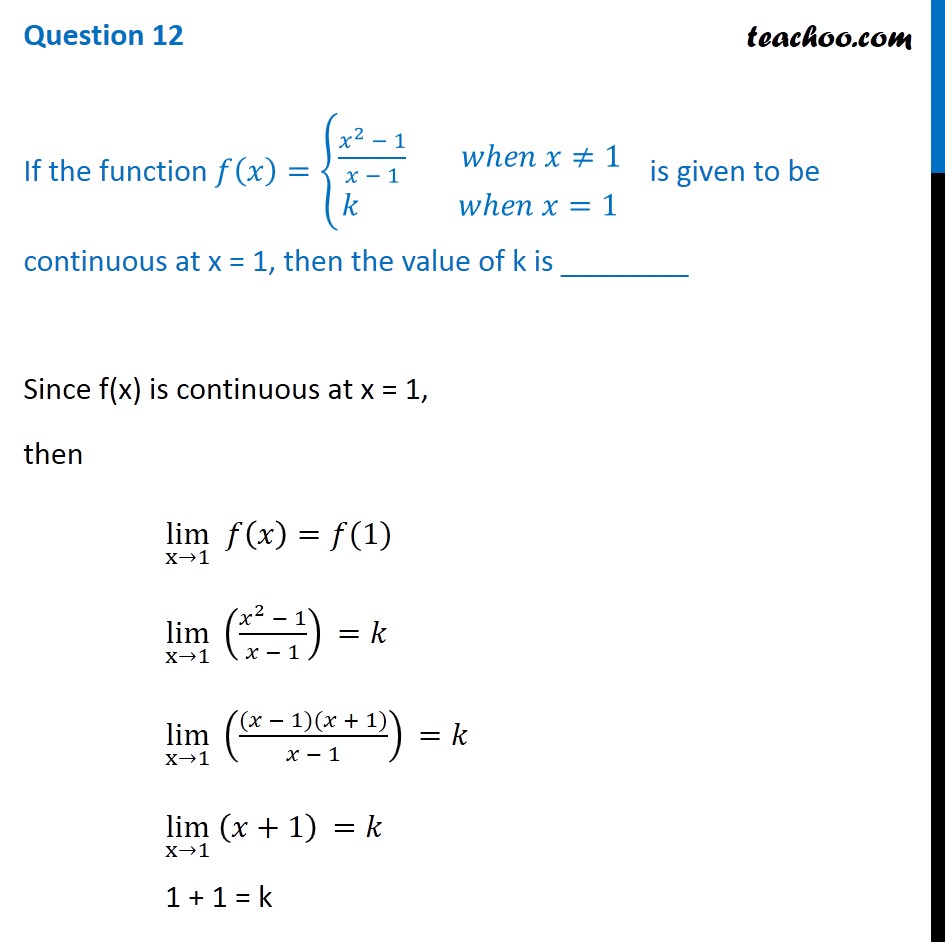



If The Function F X X 2 1 X 1 When X 1 K When X 1
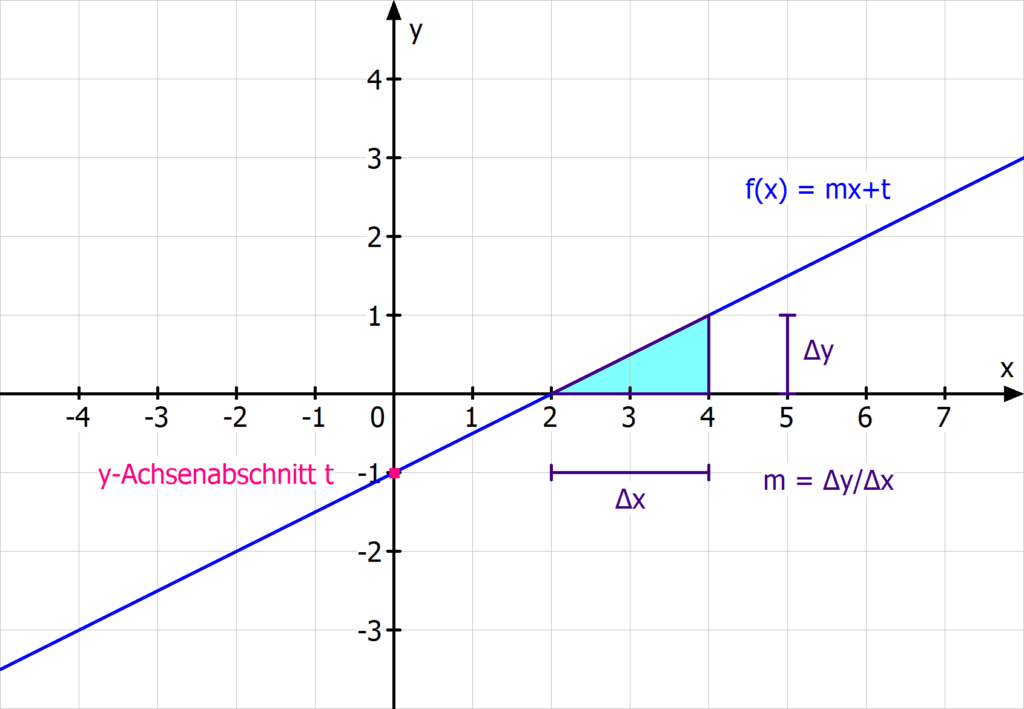



Ganzrationale Funktionen Polynomfunktionen Mit Video



What Does F X X Mean Quora



Anschauliche Bedeutung Funktionsanalyse Integral Ableitung F X Mathe Seite De




Modulus F X Geogebra




Understanding F X Function And How To Graph A Simple Function Math Algebra Graphing Functions F If 7 F If 4 Showme
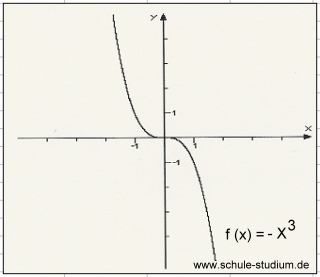



Funktionsgraphen Verschiedene Funktion X 3 X 4 Grafisch Dargestellt Schule Studium De Erklart Ausfuhrlich



An Exploration Of Combining Linear Functions
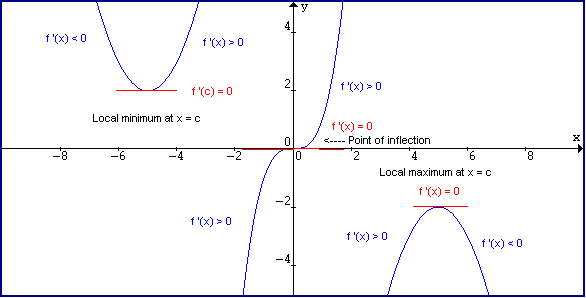



Derivative And Tangent Line
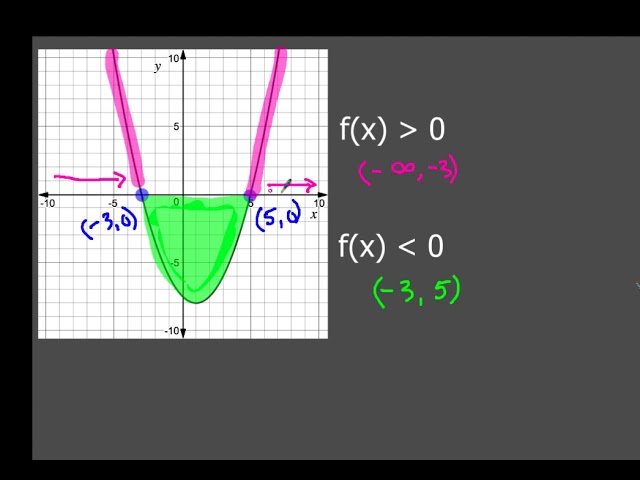



How To Tell Where F X Is Less Than 0 Or Greater Than 0 Youtube



Math Scene Equations Iii Lesson 3 Quadratic Equations



F X 1 X




Eigenschaften Von Potenzfunktionen Ubersicht
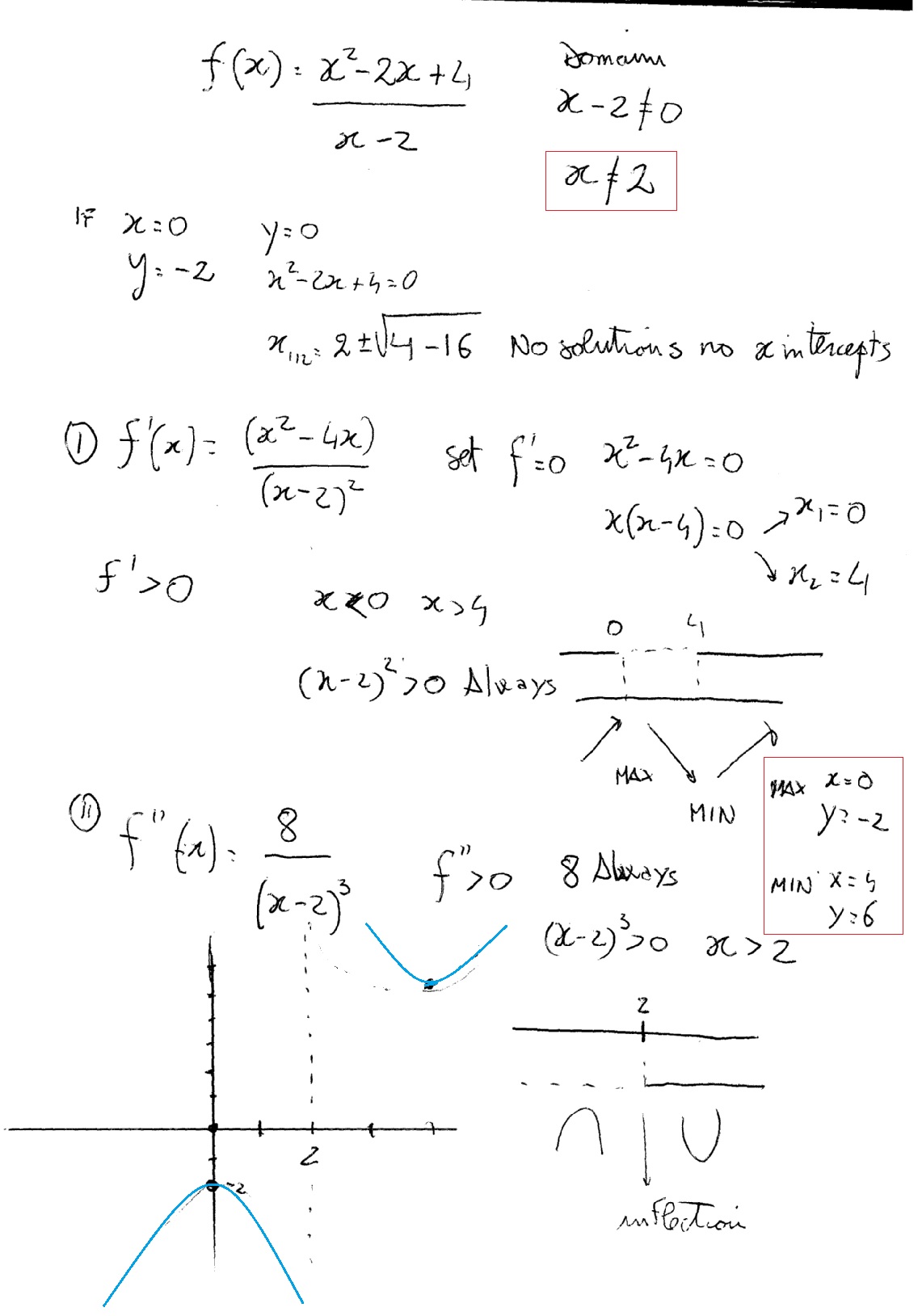



F X X 2 2x 4 X 2 F X X 2 4x X 2 2 F X 8 X 2 3 How To Find The Domain Of F X X And Y Intercepts Vertical Asymptotes The Critical Numbers Concave Up And Down And Sketch Graph Socratic



Operations On Functions Translations Sparknotes
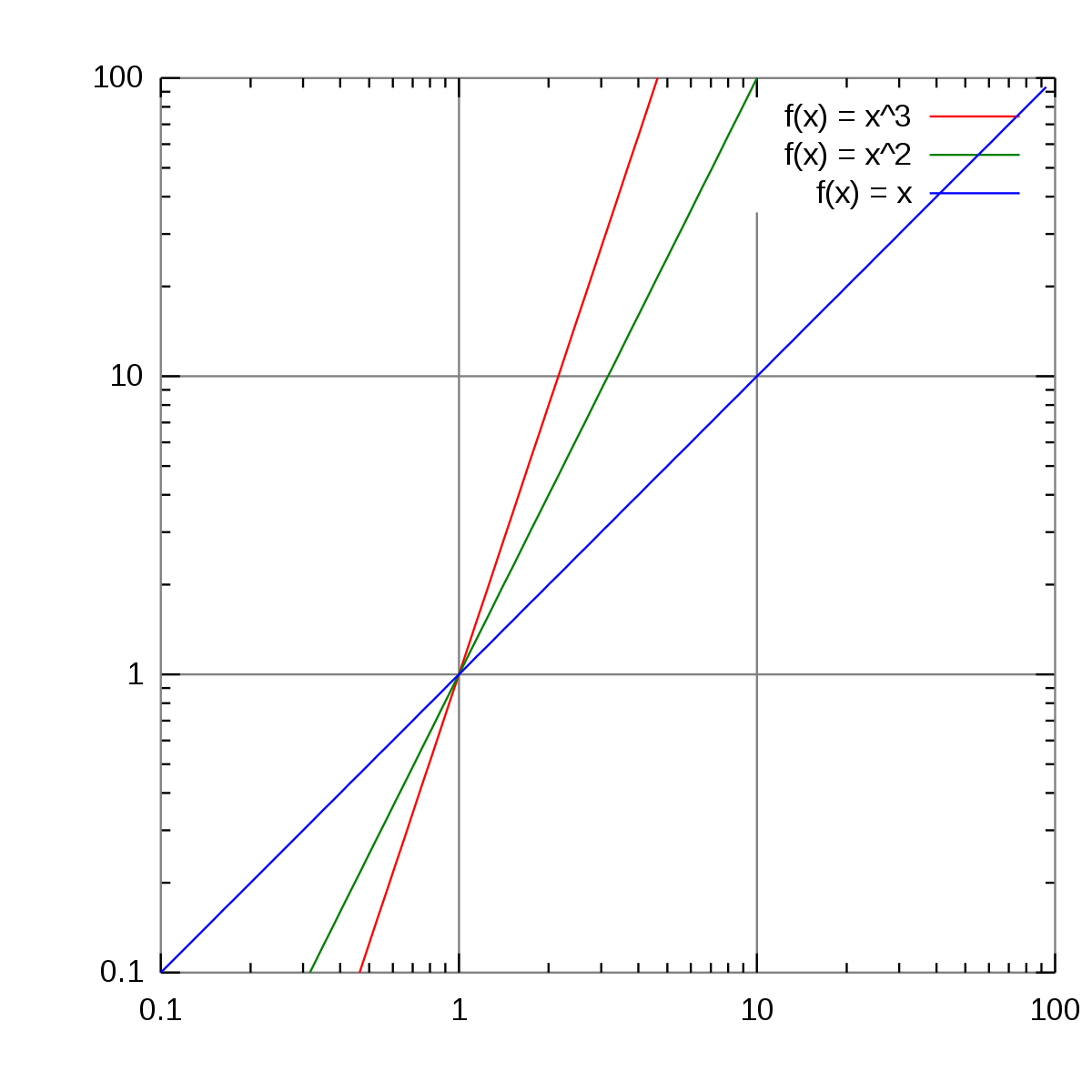



Log Log Plot Wikipedia



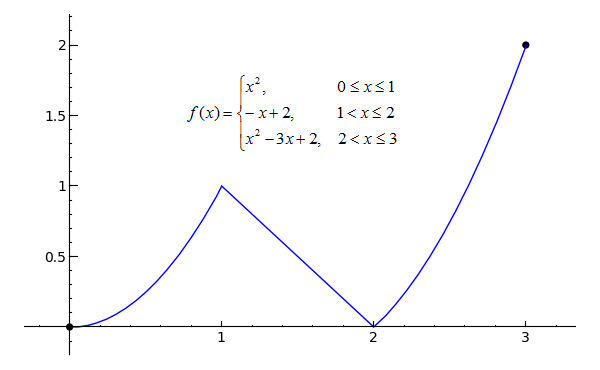
1




Fixed Point Mathematics Wikipedia
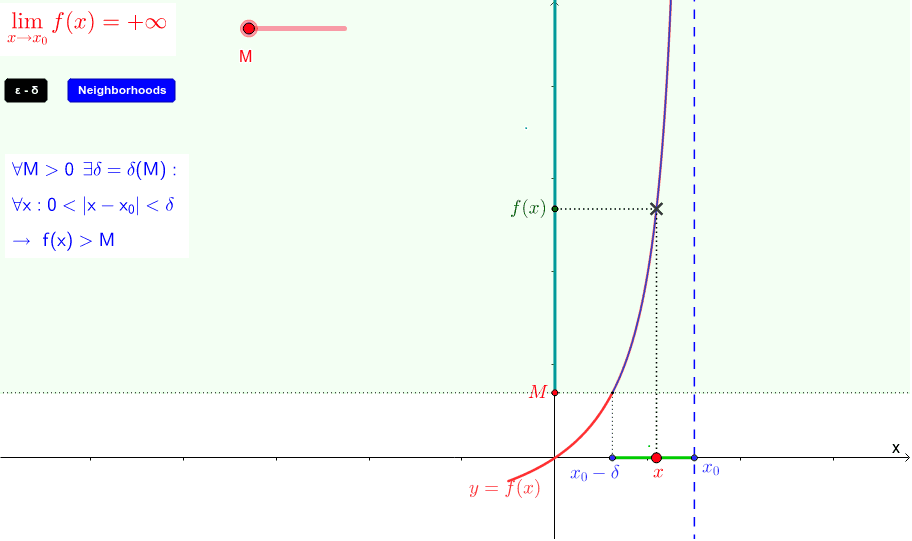



Infinite Limit Of F X When X Tends To A Finite Value Geogebra




Transformations Of The 1 X Function Video Lesson Transcript Study Com
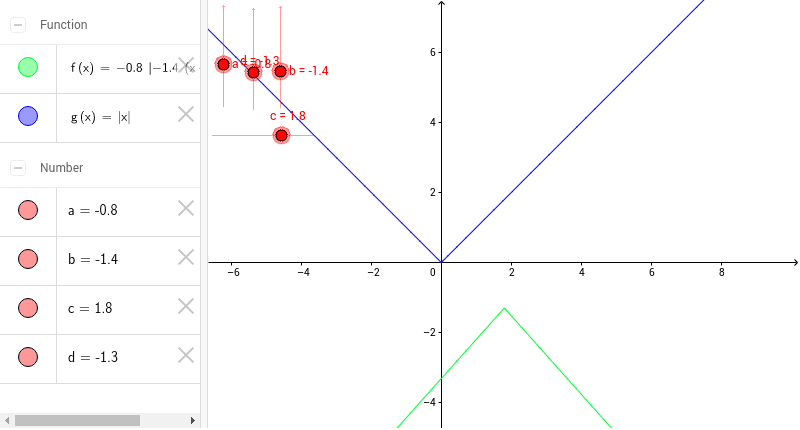



F X Lxl Geogebra



More Differentiation By First Principles



What Is The Graph Of F X X 2 Example
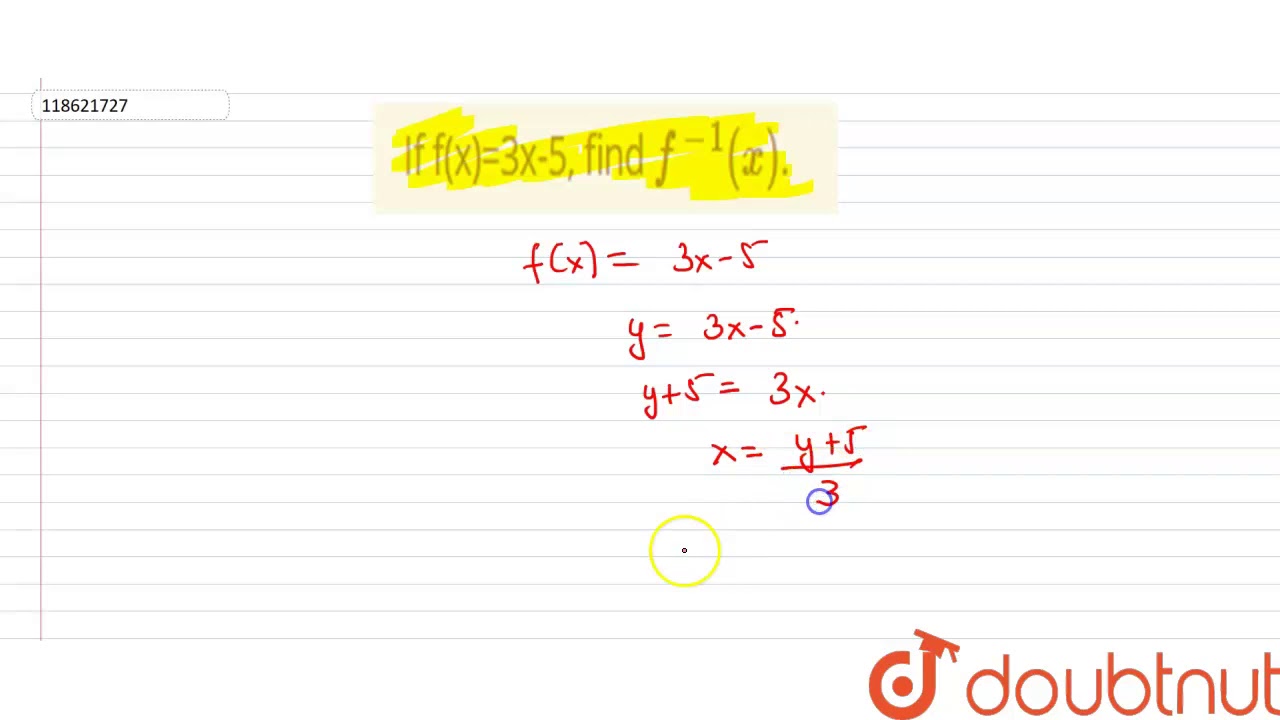



If F X 3x 5 Find F 1 X Youtube




Y F X Vertical Stretches About An Axis Ppt Video Online Download



Quadratische Funktionen Zeichnen Mathematik Klasse 10



Functions And Linear Equations Algebra 2 How To Graph Functions And Linear Equations Mathplanet
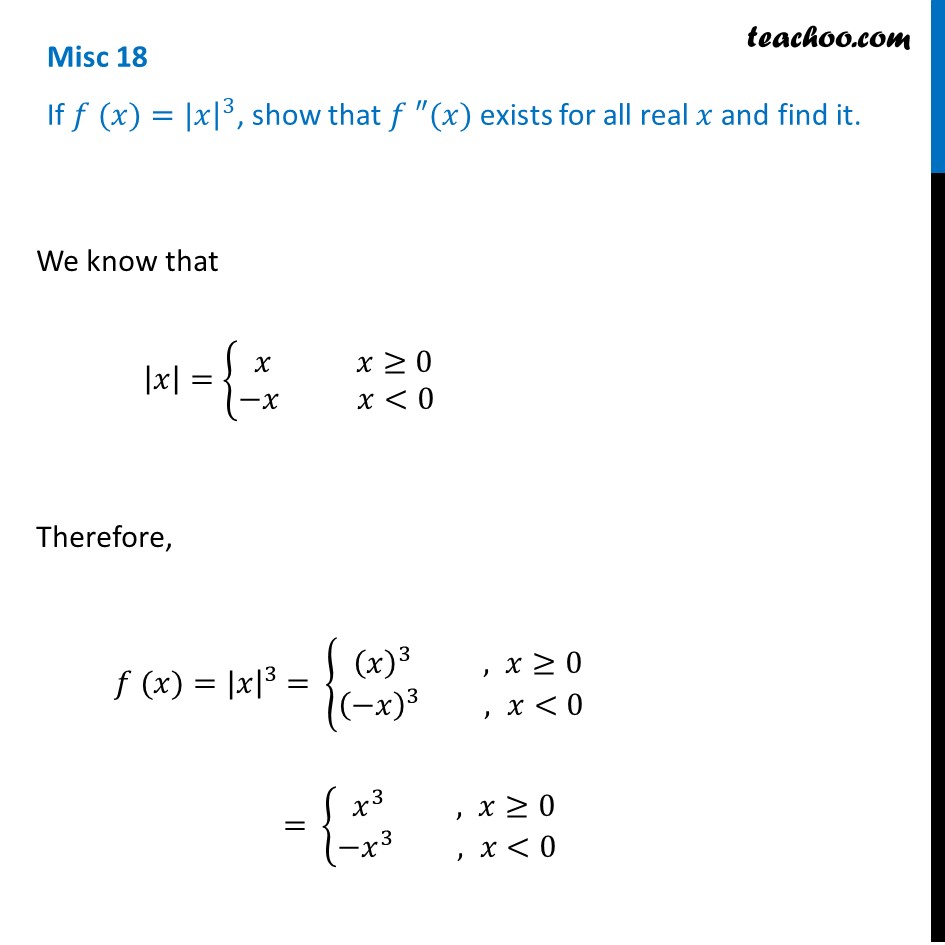



Misc 18 If F X X 3 Show That F X Exists And Find It




Obtain The Fourier Series For The Function F X X 2 Pai X Pai Mathematics 3 Question Answer Collection




Ableitungsfunktion F X Und Graphisches Ableiten Online Lernen



Sage Calculus Tutorial Continuity
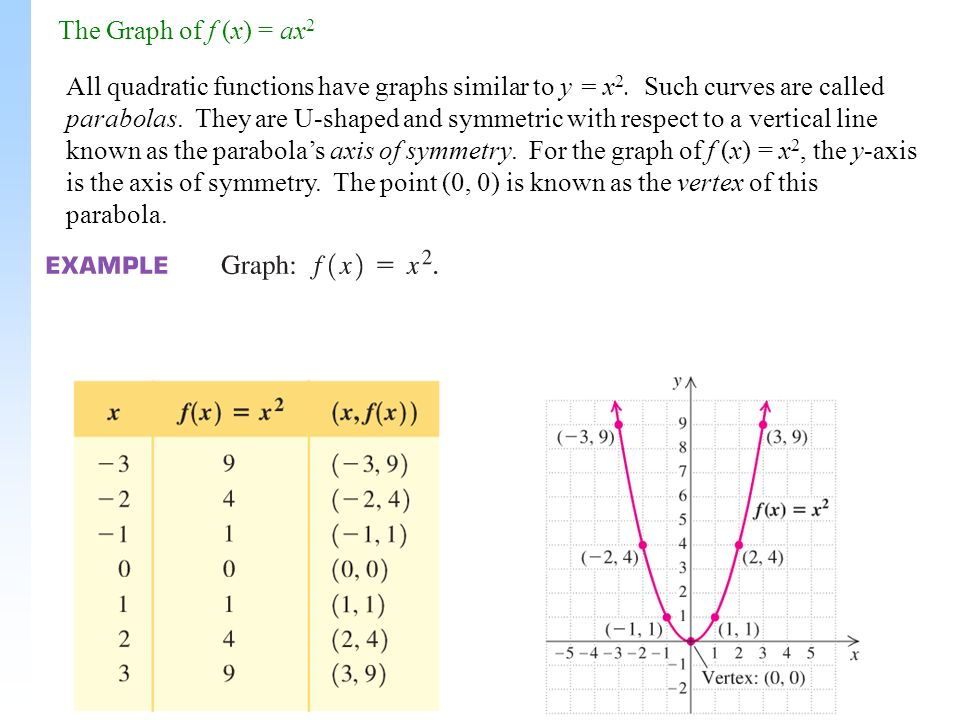



The Graph Of F X Ax 2 All Quadratic Functions Have Graphs Similar To Y X 2 Such Curves Are Called Parabolas They Are U Shaped And Symmetric With Ppt Download




Quantitative Aptitude Algebra Functions If F X 5x 2 3x 5 Handa Ka Funda Online Coaching For Cat And Banking Exams



What Does F X X Mean Quora




If F X X 2 2x 7 Find F 3



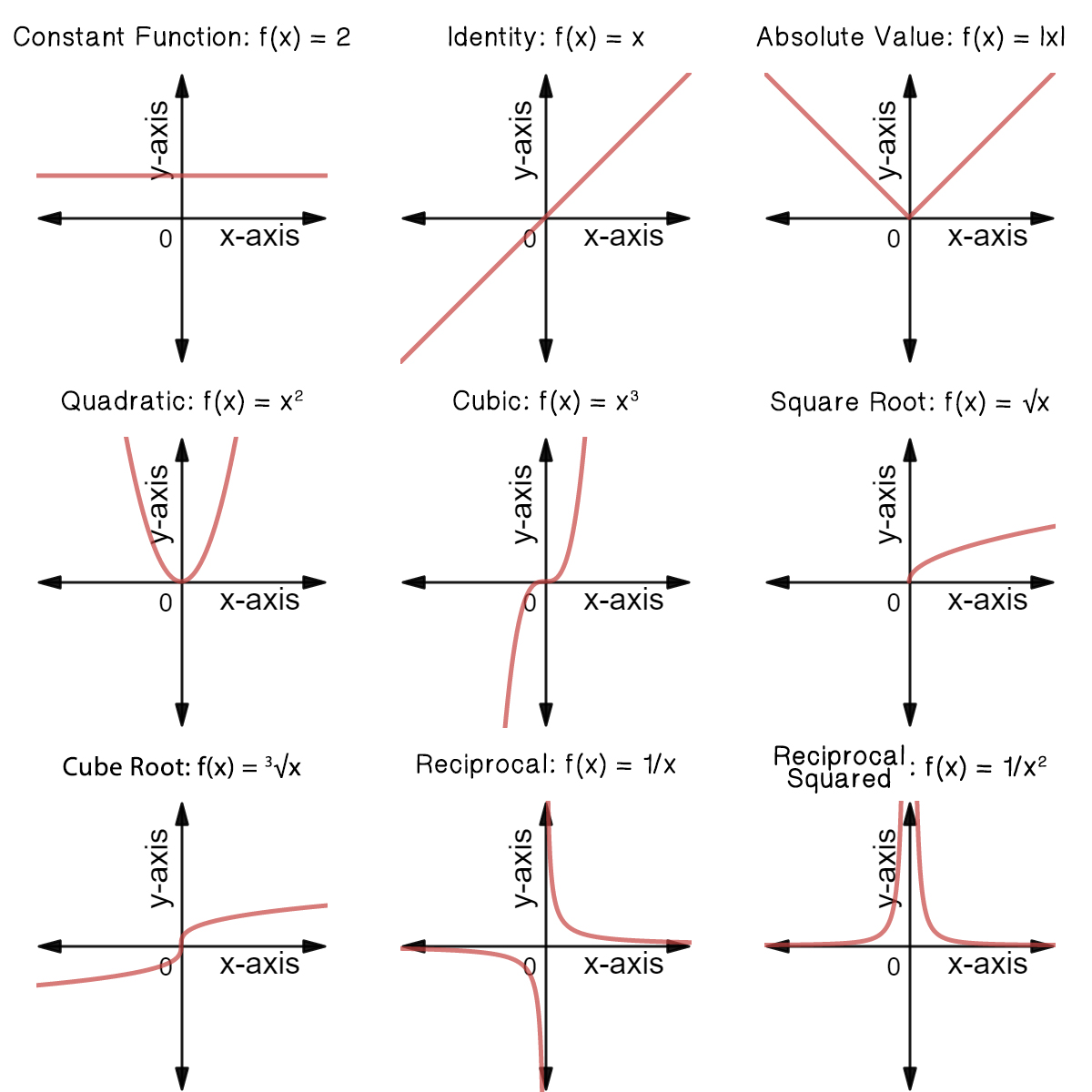
Graphing The Basic Functions



Skizzieren Des Schaubilds Der Ableitungsfunktion



Classifying Common Functions Expii




Express F X X As A Half Range Sine Series In 0 X 2 Mathematics 3 Question Answer Collection
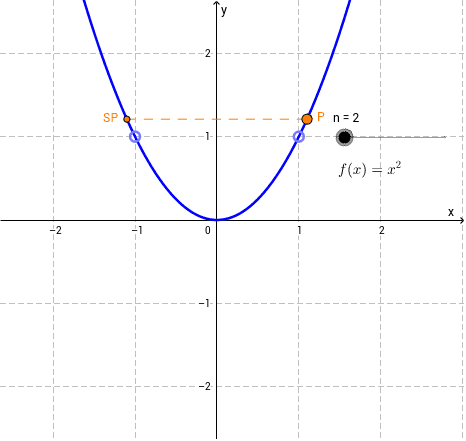



Potenzfunktion F X X N Geogebra




에프엑스 F X Facebook



Geo Ma Tum De Resources Persistent D 1 3 6 D1360b7adc5e3153afee0409a779ec1cb 10 Mehrdim Analysis Pdf



Extrempunkte Graphisch Abitur Vorbereitung
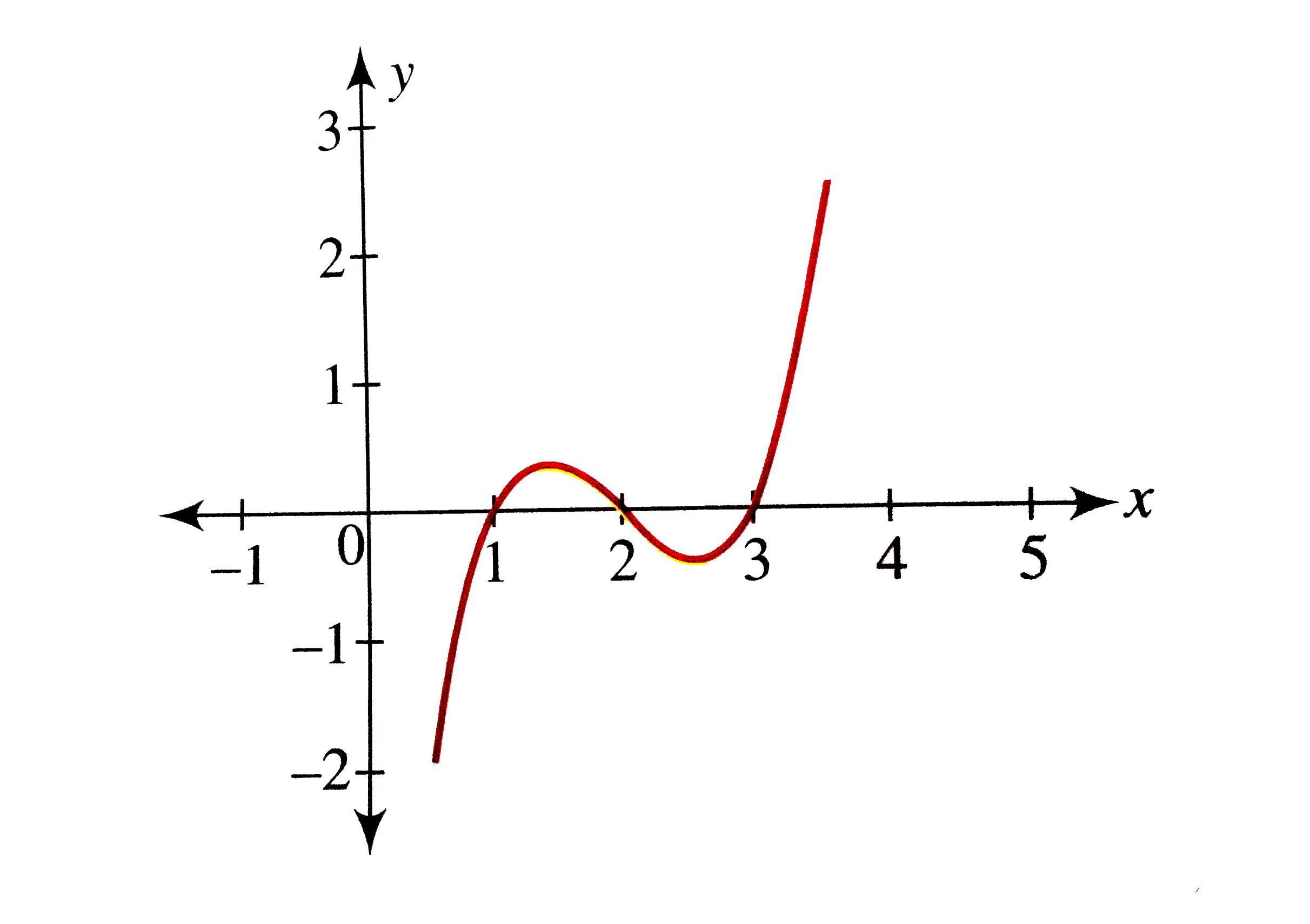



Draw The Graph Of F X X 1 X 2 X 3
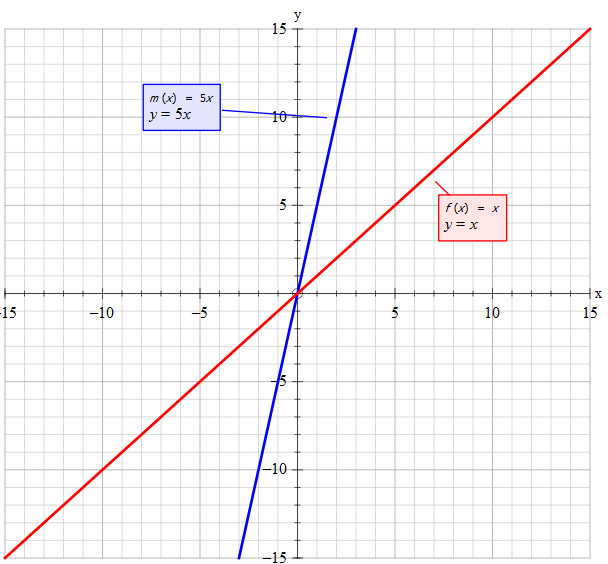



How Do You Compare The Graph Of M X 5x To The Graph Of F X X Socratic
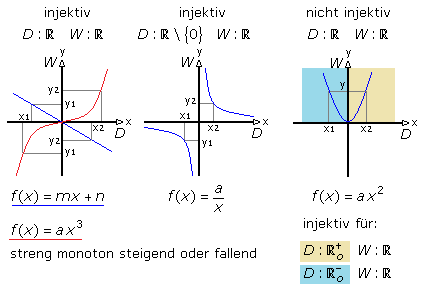



Funktion Und Umkehrfunktion




Domain And Range Of F X X 3 X 3 Are Respectively




Use Arrow Notation College Algebra



F X X Table




What Does F X X Mean Quora
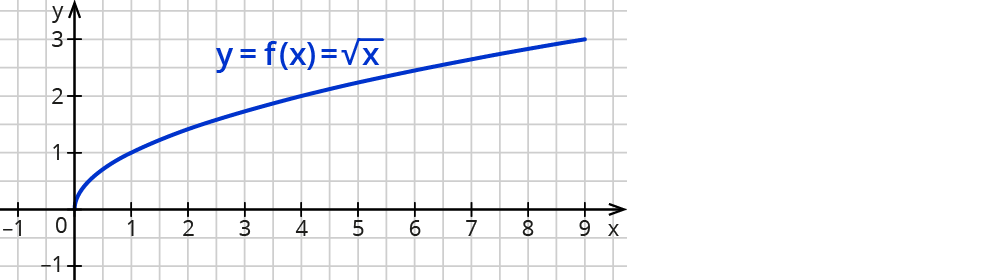



Untersuchen Der Wurzelfunktion Kapiert De



Betragsfunktion
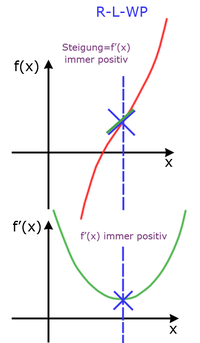



X Achse Online Lernen Auf Abiweb De Online Kurse



What S Does Y F X Mean How To Use This Powerful Six Sigma Formula




Ex 5 1 2 Examine Continuity Of F X 2x2 1 At X 3
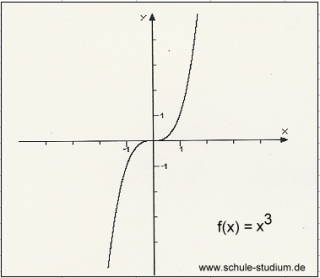



Funktionsgraphen Verschiedene Funktion X 3 X 4 Grafisch Dargestellt Schule Studium De Erklart Ausfuhrlich
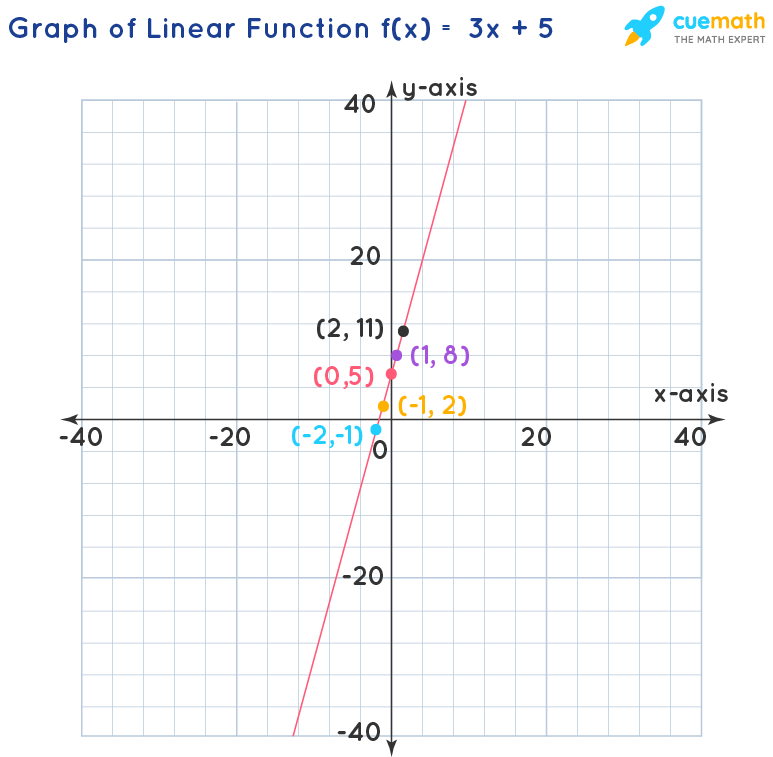



What Are The Domain And Range Of The Function F X 3 X 5 Solved




Untersuchen Der Betragsfunktion Kapiert De
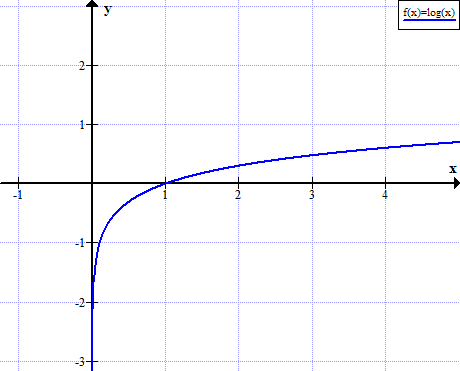



Logarithm Graph Graph Of Log X
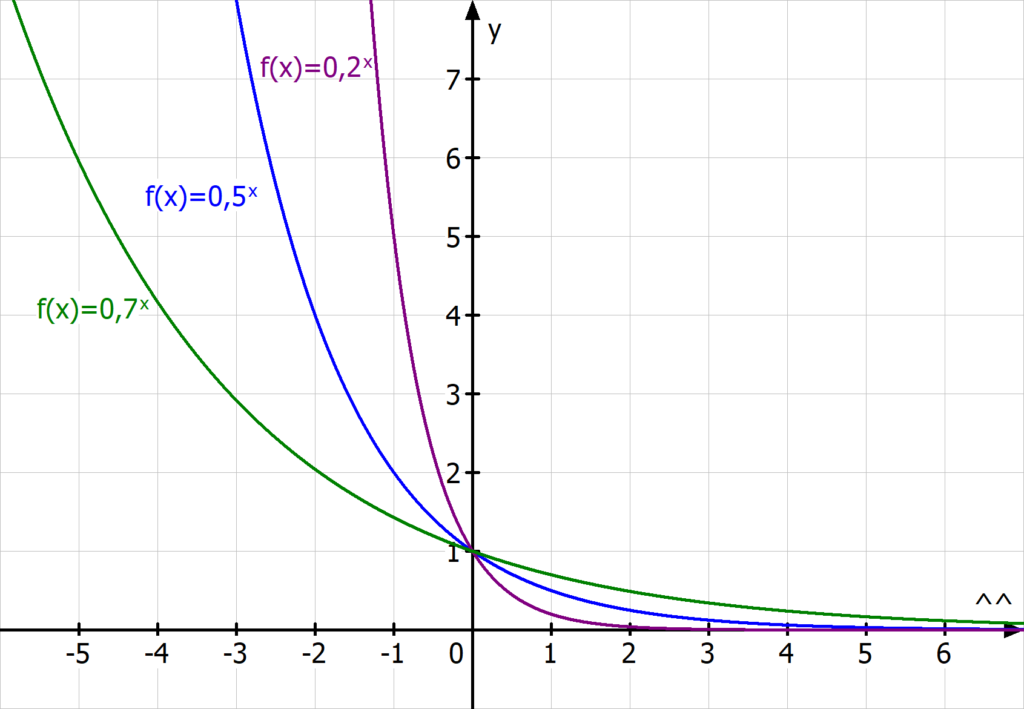



Exponentialfunktion Erklarung Beispiele Mit Video



Rational Function F X 1 X Zona Land Education



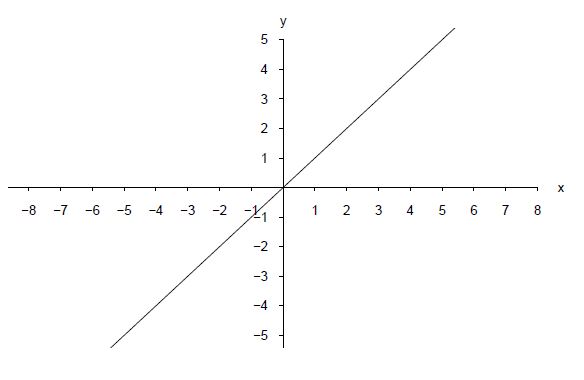
Bitte Um Zeichnung Der Linearen Funktion F X 1 Mathelounge



Graph Domain And Range Of Absolute Value Functions
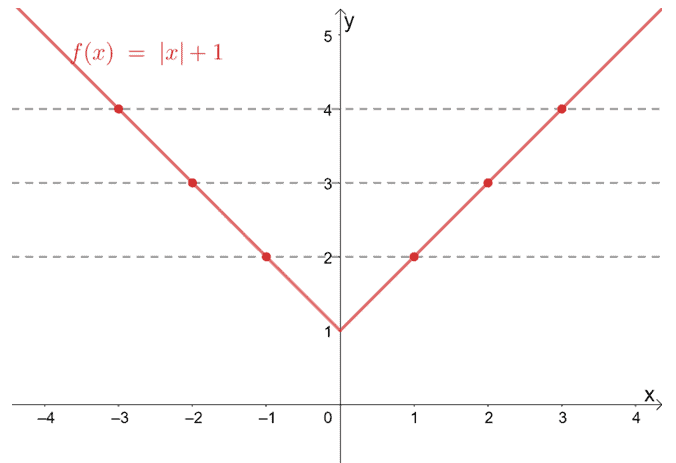



One To One Function Explanation Examples




Wertetabelle X 0 0 5 1 Y F X X 2 0 0 25 1 Graph Der Funktion Pdf Kostenfreier Download
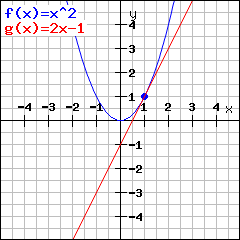



Online Derivation Calculator



Operations On Functions Reflections And Rotations Sparknotes
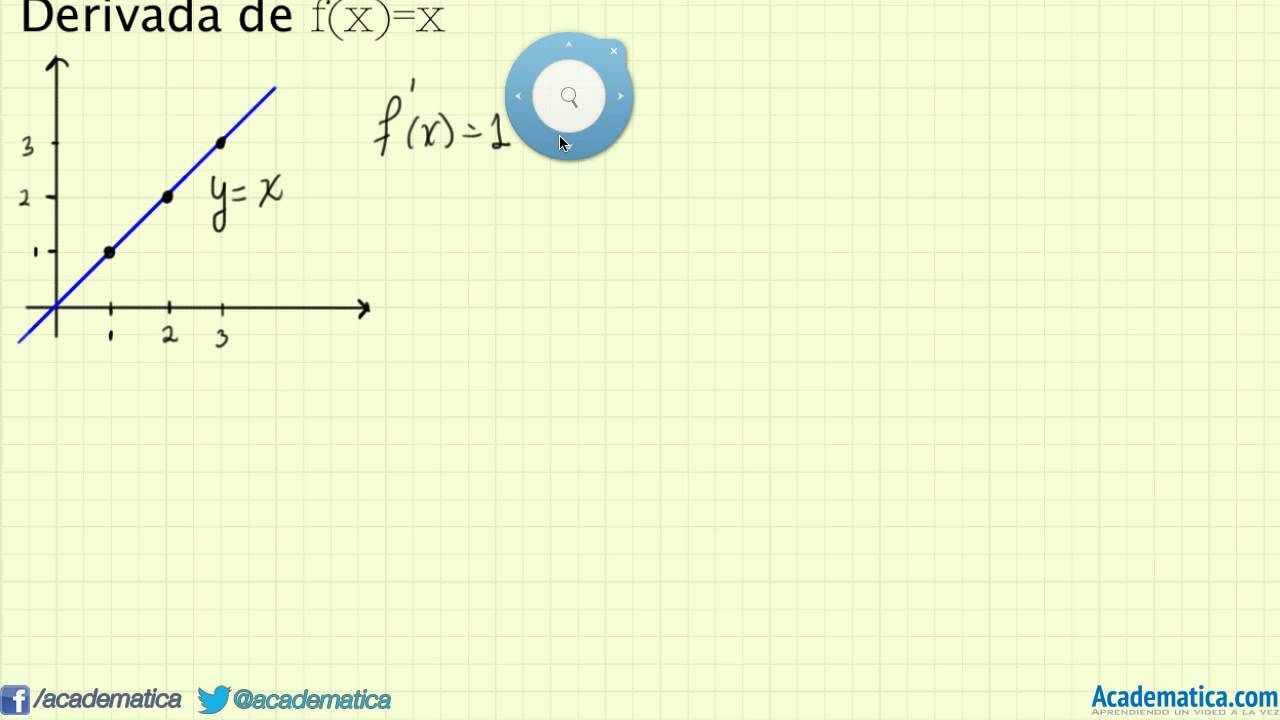



Demostracion De La Derivada De F X X Youtube



0 件のコメント:
コメントを投稿